Optical activity

Optical activity

Light interacts with matter. The interaction leads to an array of phenomena of which the most common are refraction (also called refringence) and reflection. These phenomena depend on the type of matter (e.g., metal or dielectric) and the properties of the light, such as color (= frequency), direction of propagation with respect to the material, and polarization. Any form of polarization dependence of the interaction between light and matter is called optical activity. This definition includes effects that occur without the presence of an applied magnetic or electric field (so-called natural optical activity) and polarization-dependent phenomena that occur upon placing matter in a magnetic or electric field (so-called magneto- and electro-optical activity). Two types of optical activity can be discerned: birefringence (also called double refraction) and dichroism. We define birefringence as the dependence of the speed of monochromatic light on polarization. Circular birefringence (CB) occurs when the speeds of left and right circular polarized light differ and linear birefringence (LB) when the speeds of two orthogonal linearly polarized light beams differ. CB is the most common phenomenon and gives rise to a rotation of the polarization plane of linearly polarized light (see below). In the case that these phenomena are caused under the influence of an applied magnetic field they are called magnetic circular birefringence (MCB) and magnetic linear birefringence (MLB). We define dichroism as the dependence of the absorption (or emission) of monochromatic light on polarization. Circular dichroism (CD) occurs when the absorptions (or emissions) of left and rightcircularly polarized light in matter differ and linear dichroism (LD) when the absorptions (emissions) of two orthogonal linearly polarized light beams differ. In the case that these phenomena are caused under the influence of an applied magnetic field they are called magnetic circular dichroism (MCD) and magnetic linear dichroism (MLD).

List of Contents

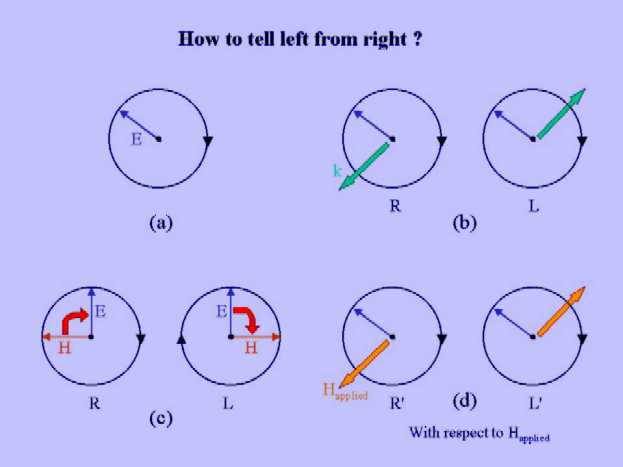
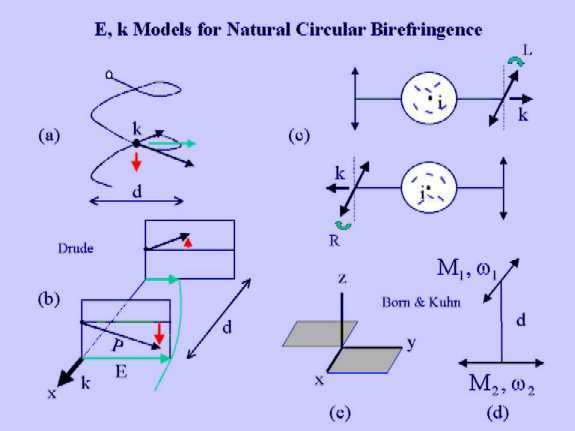
How to tell left from right?

Figure (a) depicts the time evolution of the oscillating electric field component of a circularly polarized monochromatic light beam at a fixed position in space. Can one tell from Figure (a) whether the light is left or right circularly polarized? The answer is no, one can’t! To know the sense of rotation, one needs to specify either the direction of light propagation (indicated by the k vector in Figure (b)) or the magnetic component of the electro-magnetic field (indicated in Figure (c)). From the reader’s point of view the sense of rotation of E is the same in all four diagrams in Figure (b, c). However, if the light propagates toward the reader the polarization is right circular (R) and away from the reader the polarization is left circular (L) (Figure (b)). If we consider the 90° angle between H and E in Figure (c) then we see that H rotates toward E in the case of right circular polarization and E rotates toward H in the case left circular polarization. These characterizations are intrinsic properties of light and independent of reader’s perspective. Just like the reader for telling left from right, a physical system must somehow take into account either the combination of E and k or of E and H in order to be optically active. In other words, one can discern two types of physical mechanism for explaining natural optical activity: E, k mechanisms and E, H mechanisms. The E, k mechanisms depend essentially on the spatial variation of the electro-magnetic wave along the direction k and the E, H mechanisms on an interplay of electric polarization and magnetic induction. A more detailed mechanism for either one will be specified further on. In the presence of an applied magnetic (or electric) field one should think of the physical system as "matter plus field". So defined, the system provides a unique spatial direction of reference (namely the field) for unambiguously assigning a rotational sense to the rotating electric field E. 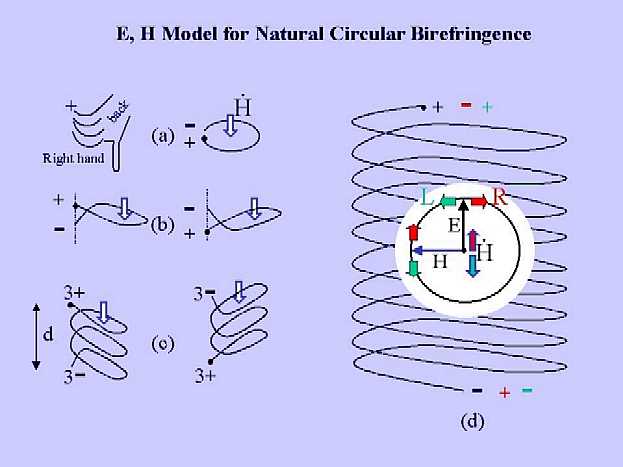The rotational sense defined with respect to the applied field is indicated by R' or L' in Figure (d). The sense defined with respect of the field can either coincide or be opposite to the rotational sense of the radiation (the latter being defined with respect to k). Magneto-optical effects involving circular or elliptic radiation depend essentially on the distinction between R' and L' and not on that between L and R (see below).

List of Contents

Mechanisms for natural circular birefringence

E, k models

In 1874 Ludwig Boltzmann published a paper concerning "the relation between the rotation of the polarization plane and the wavelength of different colors" in which he criticized an expression for the dispersion relation given by von Lang and Stefan. Therein Boltzmann stated: It seems to me probable on theoretical grounds, that the angle of rotation of the plane of polarization could be expressed better by a formula of the form

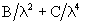than by the formula

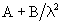. Boltzmann based his critique on the argument that "The rotation of the plane of polarization is one of those phenomena which depend on the fact that the wave lengths are no longer very large compared with the sphere of action of a molecule". Indeed, the rotation approaches zero in the long wave length limit according to the expression proposed by Boltzmann. The discussion is a prelude to the development of E, k models for natural circular birefringence.
The first quantitative theory for natural circular birefringence was formulated by Drude in his "Lehrbuch der Optik" that was published in 1900. Drude started has analysis by comparing a chiral molecule with a cylindrical coil with diameter d (Figure (a, b)). The molecule was assumed to contain a single charged particle (electron) whose motion was confined to the coil. Subsequently, the system was placed in a beam of polarized light incident normal to the axis of the coil (the light propagates toward reader in Figure (a)), and the interaction of the electric field component of the radiation field (E in Figure (a, b)) on the charged particle was analyzed. Although Drude’s analysis has been criticized in later work (see below), it is of interest to follow some of his arguments. A cornerstone of Drude’s theory is that there exists a non-local interaction between the radiation field and charged particles. Let us assume that the incident wave is linearly polarized as indicated in Figure (a). A perspective view of Figure (a) is presented in Figure (b). It shows the electric field (green arrows) at two diametrical points of the coil along the k vector. The electric force acting on the particle leads to displacements (P) along the coil. The displacements have two components, one along the E and one perpendicular to E (Figure (b)). The perpendicular components are due to the spatial configuration of the coil (see Figure (a)) and have opposite signs in the planes in the front and the back (Figure (b)). The fields, however, are slightly different in size due to the spatial progression of the phase (a section of the electric wave has been indicated by a green curve in Figure (b)). As a consequence, the perpendicular forces in the two planes have different magnitudes and do not cancel. According to the concept of "non-local interaction" the particle interacts simultaneously with the electric fields at the two diametrical points and undergoes a net perpendicular displacement, which is the sum of the displacements in the font and back plane (red arrow in Figure (a)). Of course, polarization of a dielectricum perpendicular to the polarization plane of the incident radiation is required for a rotation of the polarization plane to occur. For a rotation, however, the perpendicular displacement should be in phase with the displacement parallel to E. The perpendicular displacement, however, is proportional to the derivative of the electric field, that is, 90º out of phase compared to the parallel displacement. Thus, there arises an elliptical distortion of the wave (CD), and not a rotation (CB). A complete mathematical analysis of the Drude model, given by Kuhn (Zeitschrift für physikalische Chemie, 20 (1933) 325), supports the conclusion that this model does not yield circular birefringence. The theoretical expression for the CB derived by Drude agrees, however, closely with that given by Kuhn (see below), indicating that the original treatment does not apply to the physical model given in Figure (a, b). Born criticized the Drude model on several grounds. The Drude model is essentially a classical theory in which matter is built from point like particles. From a classical perspective the origin of the non-local interactions is obscure. This concept seems to fit better into a quantum mechanical description of the problem where one is dealing with spatially delocalized states. The classical description of matter as an ensemble of elastically bound charged particles has been successfully applied to the interpretation of a variety of phenomena, including the dispersion of the refractive index, the Zeeman effect, and the Faraday effect. Along similar lines, a theory for natural CB has been proposed by Born (Physikalisch Zeitschift XVI (1915) 251). An ensemble of harmonic oscillators, however, is optically inactive, even if the oscillators are anisotropic. This point is illustrated in Figure (c). The diagram in the upper panel shows a plane polarized light beam traversing a medium containing harmonic oscillators (small blue sticks). Suppose there would be a rotation (L). Inversion (i) yields the diagram in the lower panel of Figure (c). The oscillators and the rotation from reader’s point of view are not changed by the inversion, but the wave vector has flipped its sign. Thus, the intrinsic sense of the rotation is reversed to R. The upper and lower panel represent, however, the same physical situation, hence a = -a and the rotation is zero. To overcome this difficulty, Born introduced the concept of interacting harmonic oscillators. Figure (d) shows the simplest realization of an optically active system based on interacting harmonic oscillators, proposed by Kuhn. The potential energy is given by

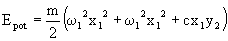
(1)

(M₁ = M₂ = m). The requirements for chirality are the following: (1) system is non-planar, (2) the coefficient c is different from zero. The first point implies that d ¹ 0 and that the two oscillators are not coplanar. The interaction potential between the two oscillators is positive in the gray areas shown in Figure (e) and negative otherwise and confers on the system a "handiness" as of a coordinate frame. An analytical expression for the rotatory dispersion of this theoretical model was derived by Kuhn. The realization that at least two harmonic oscillators are required for circular birefringence to occur has deflected the attention from alternative approaches for quite a while. As we shall see in the next section, the requirement of there being two or more oscillators can be dropped if one considers particles moving in an anharmonic potential.

List of Contents

E, H models

The prototype of an E, H model was formulated by Rosenfeld (Zeitschrift für Physik 52 (1928) 161). The final result of his lengthy quantum-mechanical derivation was the following simple expression for the rotational angle of the polarization plane for a randomly oriented sample of molecules

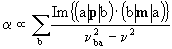
(3)

The rotation depends on a scalar product of the electric and magnetic transition momenta, the summation being over the electronic transitions in the molecule. The consideration of both the electric and magnetic field components of the radiation field distinguishes the present theory from theories that consider exclusively the electric field component of the radiation field (see E, k Models). The physical basis of Rosenfeld’s expression can readily be appreciated from an analysis of the combined effect of the electric and magnetic components of the radiation field on Drude’s coil (Figure a-d). Figure (a) shows the charge separation at the extremities of a single coil loop due to magnetic induction (the time derivative of H is indicated by a dot). Figure (b) indicates how these charges can give rise to an electric dipole of either sign by making appropriate displacements of the extremities. Figure (c) illustrates how the electric dipole increases with the number of loops; the sign of the dipole depends on whether one is dealing with a right handed or left handed screw. Notice that there is (1) a proportional increase in the charge collected at the coil extremities with the number of loops and (2) a proportional increase in the charge separation (which depends on the pitch of coil). The quantity relevant to (1) is the product of loop surface and loop number and can be identified with the magnetic dipole moment (m) of the coil. The charge separation under (2) is related with the electric dipole moment (p) of the molecule. The product of the two quantities gives the electric polarization of the molecule arising from magnetic induction and is the basis of Eq 3. Finally, Figure (d) illustrates how the rotational sense of circularly polarized light affects the net electric polarization of the coil. The electric field E leads to accumulation of positive charge at the top the coil and to negative charge at the bottom (charges indicated in black), irrespective of the sense of rotation of E. On the contrary, the rotational sense does affect the polarity of the induction dipole. Realizing that

, it follows that L and R rotations (indicated by bold arrows) give rise to oppositely signed derivatives

. Accordingly, the induction dipole associated with R circular polarized light is aligned antiparallel (see charges indicated in red) to the electric-field induced dipole, leading to a reduction of the charge polarization along the coil axis. The induction dipole associated with L circular polarized light is aligned parallel (see charges indicated in blue) to the electric-field induced dipole, leading to an increase of the charge polarization. As the refractive index is an increasing function of the polarization, we obtain n_L > n_R in the example of Figure (d). This result implies that c_L < c_R and leads to a right rotation (for an observed looking into the beam) of the polarization plane when the coil in Figure (d) is traversed by linearly polarized light. Of course, the sign of the rotation is reversed if the coil is replaced by its mirror image.
Representing a molecule by a coil, as it was done in the foregoing discussion, may seem unrealistic. Nonetheless, the example suggests that coupled oscillators are not a condition sine qua non for natural CB. Eq 3 has been used by Condon et al. for calculating the CB of a single particle moving in an anharmonic potential of the form

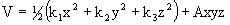The study demonstrated convincingly that a single electron moving in a field of suitable dissymmetry can give rise to optical rotatory power in a medium containing molecules of this type. Eq 3 formulates CB as a one-electron operator and is therefore ideally suited for calculating rotatory power from molecular orbital theories. Such applications have been reported by Kauzmann et al.

List of Contents